To get a human or AI tutor to help you, click Register
Sample Question 17:
Step 1: We know the area of the five unit squares is 5 so the shaded area must be 2.5. Additionally, if we were to add a unit square to the shaded area, we'd create a triangle with an area of 3.5.
Step 2: Using this information, we can infer the equation \((3-c) * 3 = 7\), which gives us the area of the would-be triangle. We can solve this equation for \(c\).
Step 3: Re-arranging the equation, we get \(3-c=\frac{7}{3}\), then we subtract to isolate \(c\), yielding \(3-\frac{7}{3}=c\).
Step 4: Simplifying, we get \(3-\frac{7}{3} = \frac{9-7}{3} = \frac{2}{3}\).
Step 5: Hence, \(c=\frac{2}{3}\) which corresponds to answer choice (C).
Five unit squares are arranged in the coordinate plane as shown, with the lower left corner at the origin. The slanted line, extending from \((c,0)\) to \((3,3)\), divides the entire region into two regions of equal area. What is \(c\)? 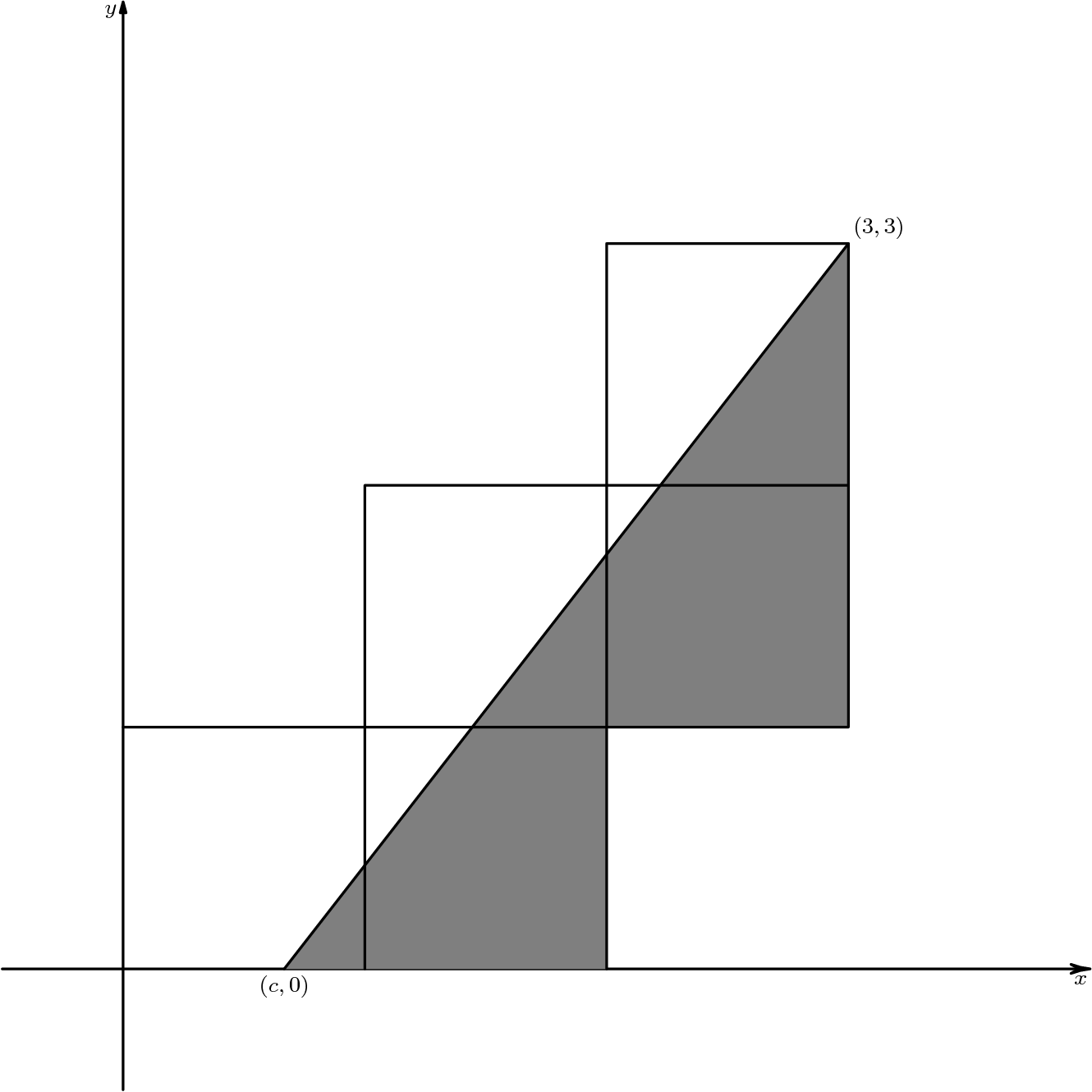
\(\text{(A) } \frac 12 \qquad \text{(B) } \frac 35 \qquad \text{(C) } \frac 23 \qquad \text{(D) } \frac 34 \qquad \text{(E) } \frac 45\)
Answer Keys
Question 17: CSolutions
Question 17Step 1: We know the area of the five unit squares is 5 so the shaded area must be 2.5. Additionally, if we were to add a unit square to the shaded area, we'd create a triangle with an area of 3.5.
Step 2: Using this information, we can infer the equation \((3-c) * 3 = 7\), which gives us the area of the would-be triangle. We can solve this equation for \(c\).
Step 3: Re-arranging the equation, we get \(3-c=\frac{7}{3}\), then we subtract to isolate \(c\), yielding \(3-\frac{7}{3}=c\).
Step 4: Simplifying, we get \(3-\frac{7}{3} = \frac{9-7}{3} = \frac{2}{3}\).
Step 5: Hence, \(c=\frac{2}{3}\) which corresponds to answer choice (C).