To get a human or AI tutor to help you, click Register
Sample Question 7:
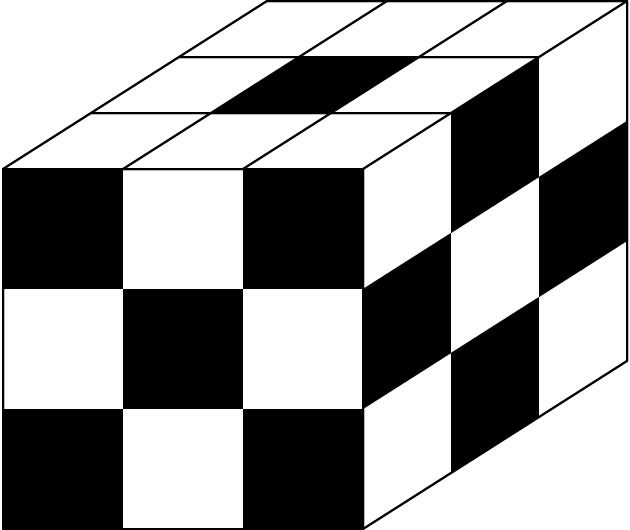
Step 1: Observe that no unit cube has more than one face painted. Hence, the number of unit cubes with at least one face painted is equal to the number of painted unit squares.
Step 2: Count the number of painted unit squares on the half of the cube that is visible in the figure, which should be 10.
Step 3: Since the opposite face is shaded the same way, multiply the count from Step 2 by two to account for the cubes on the hidden half of the cube. This gives us \(10 * 2 = 20\).
Step 4: Conclude that there are 20 unit cubes with at least one face painted. The answer is \(\boxed{\text{C}}\).
The large cube shown is made up of \(27\) identical sized smaller cubes. For each face of the large cube, the opposite face is shaded the same way. The total number of smaller cubes that must have at least one face shaded is
\(\text{(A)}\ 10 \qquad \text{(B)}\ 16 \qquad \text{(C)}\ 20 \qquad \text{(D)}\ 22 \qquad \text{(E)}\ 24\)
Answer Keys
Question 7: CSolutions
Question 7Step 1: Observe that no unit cube has more than one face painted. Hence, the number of unit cubes with at least one face painted is equal to the number of painted unit squares.
Step 2: Count the number of painted unit squares on the half of the cube that is visible in the figure, which should be 10.
Step 3: Since the opposite face is shaded the same way, multiply the count from Step 2 by two to account for the cubes on the hidden half of the cube. This gives us \(10 * 2 = 20\).
Step 4: Conclude that there are 20 unit cubes with at least one face painted. The answer is \(\boxed{\text{C}}\).